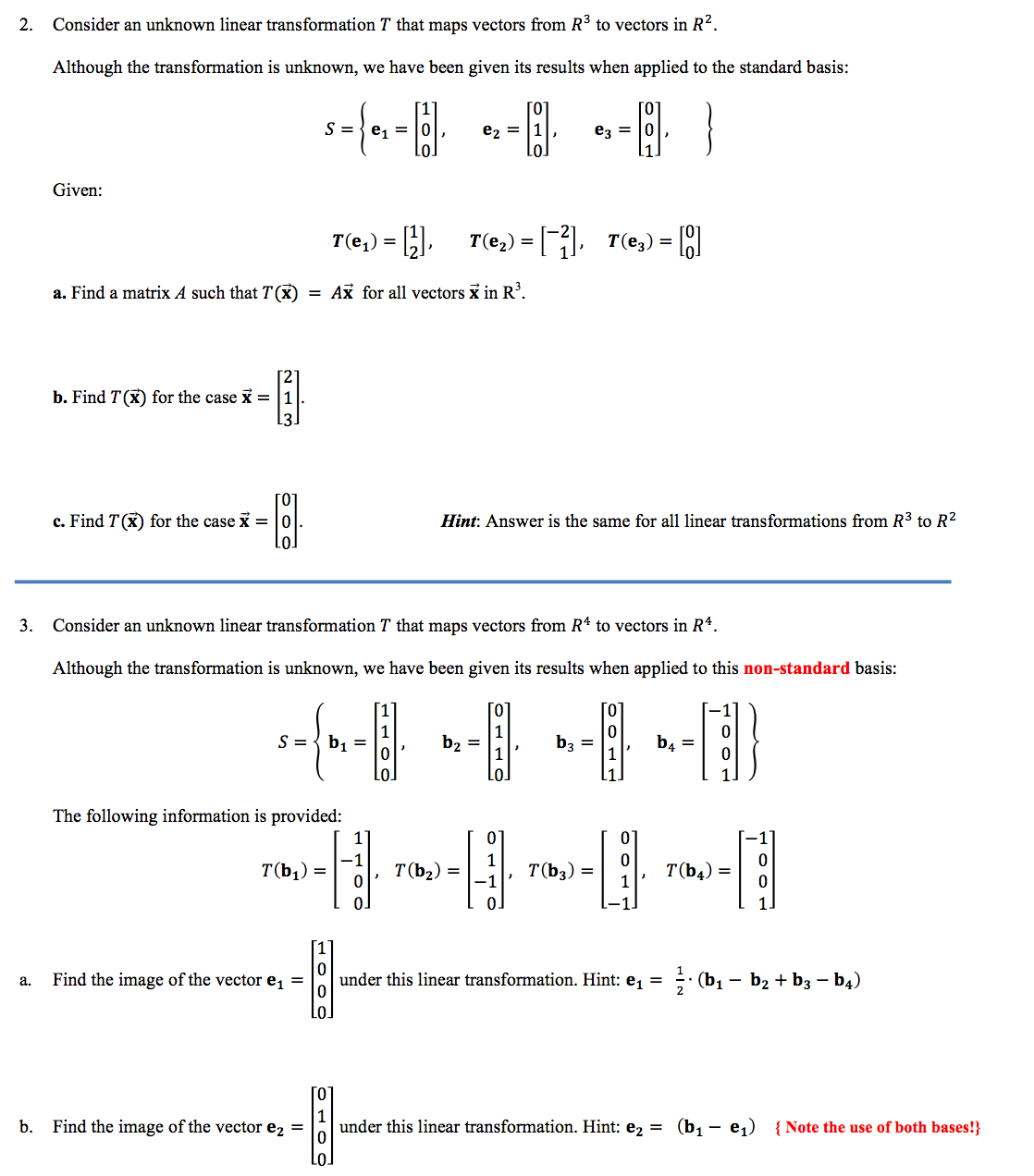
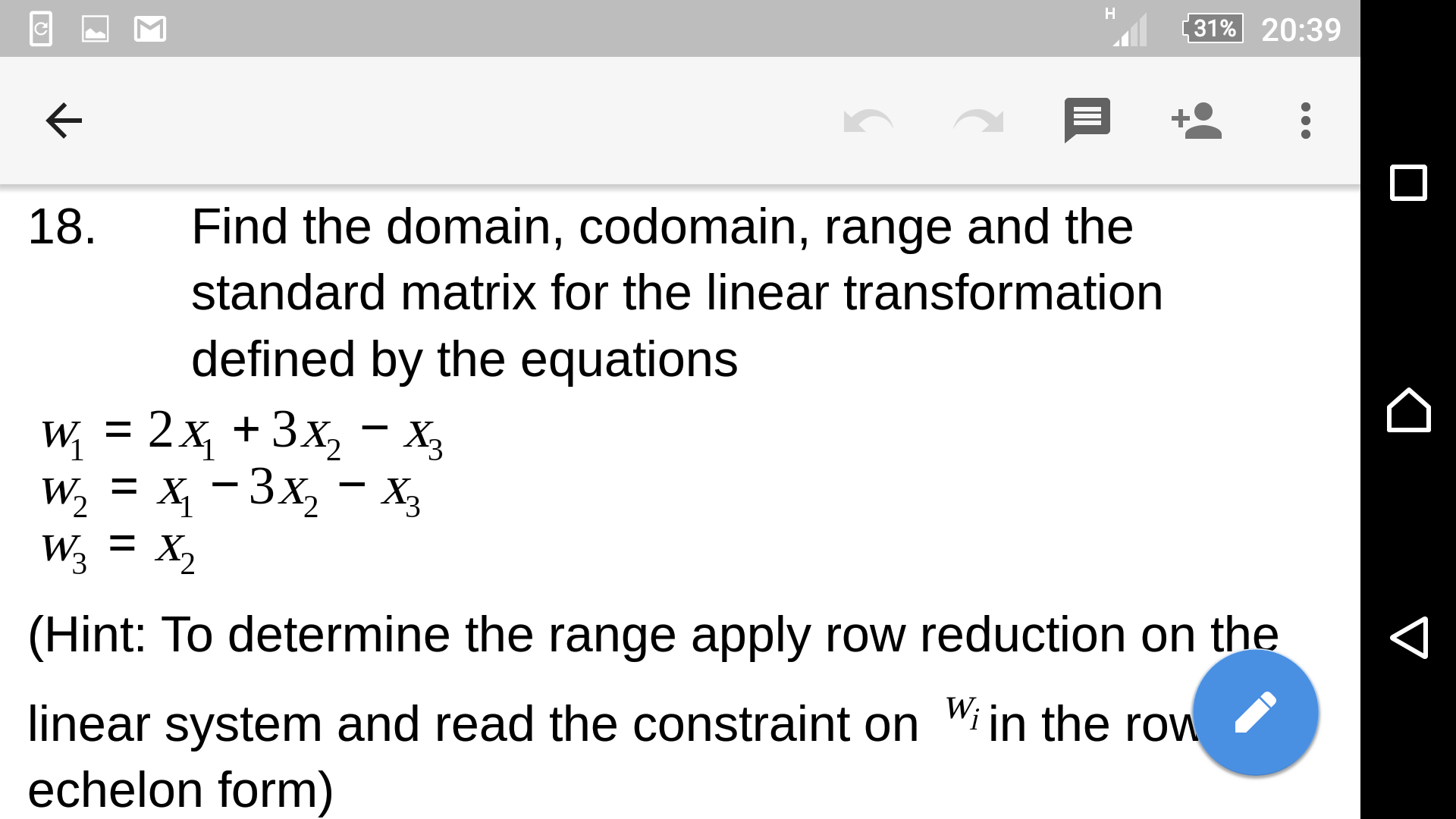
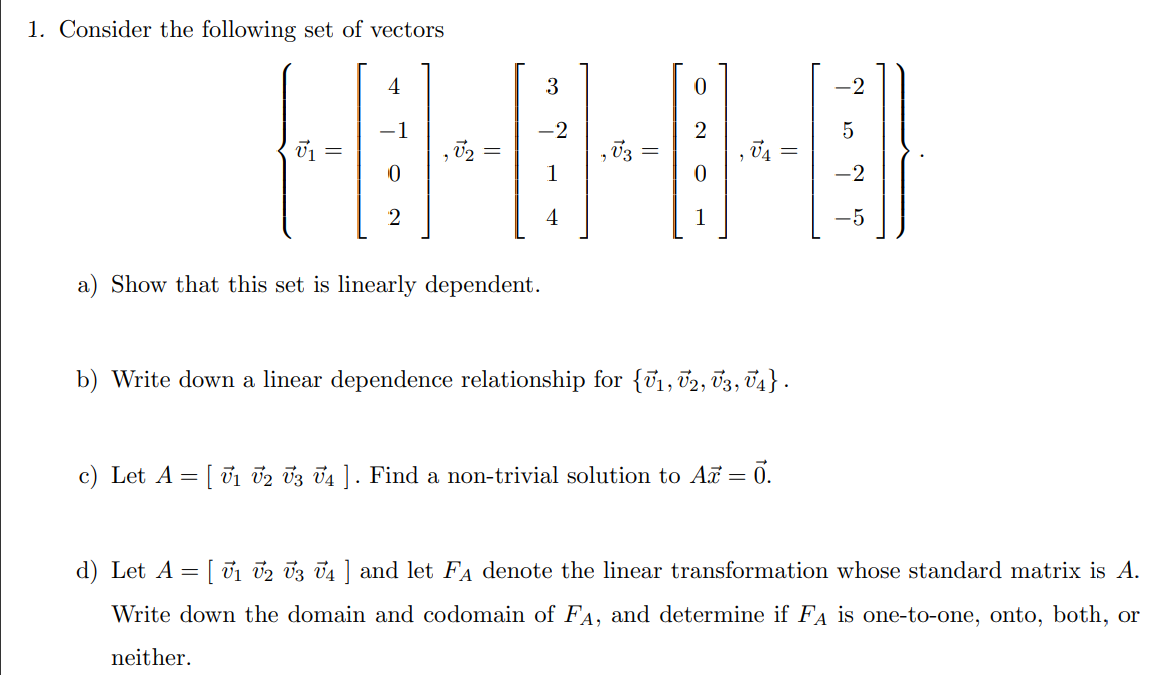
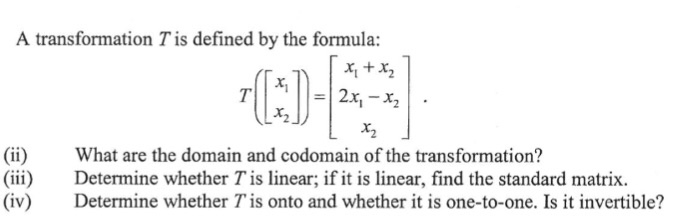
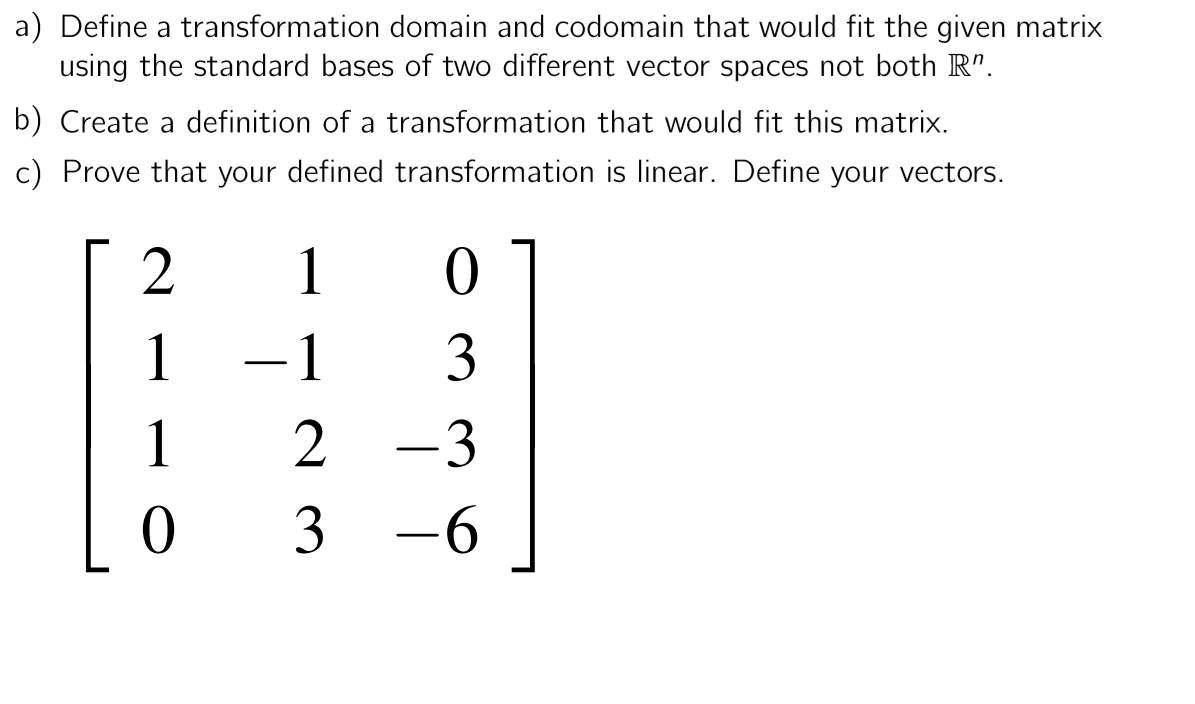
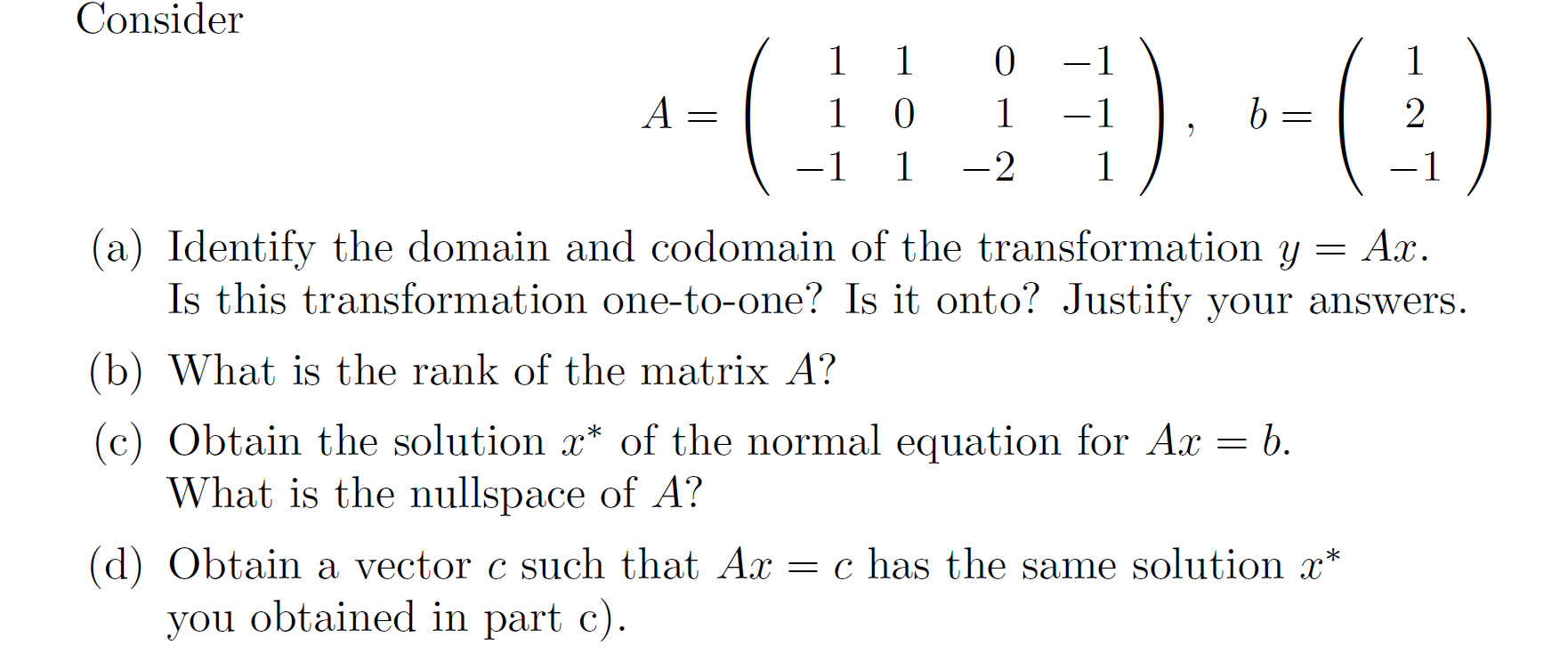
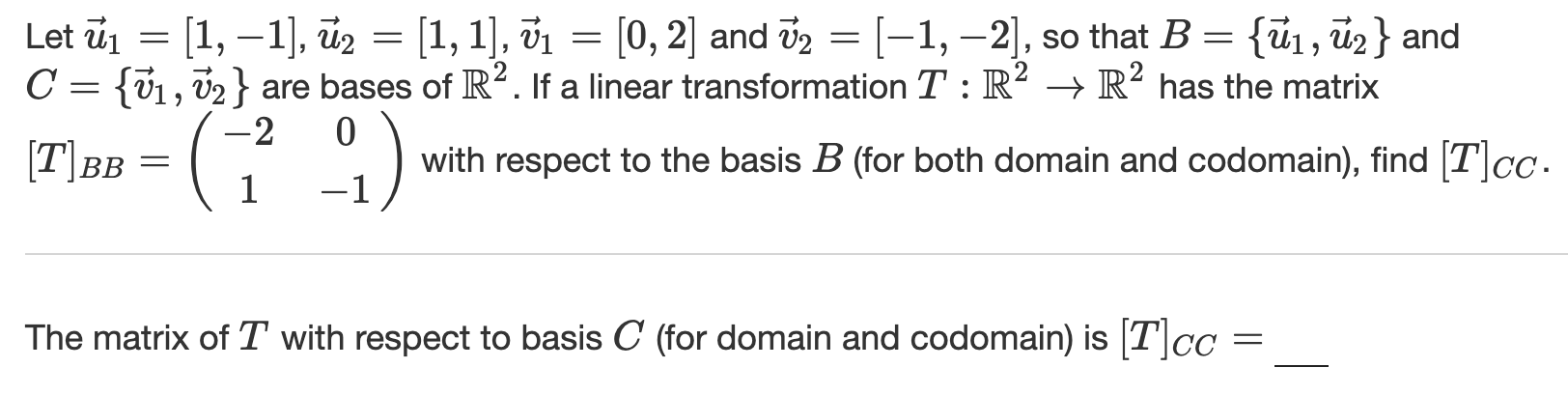
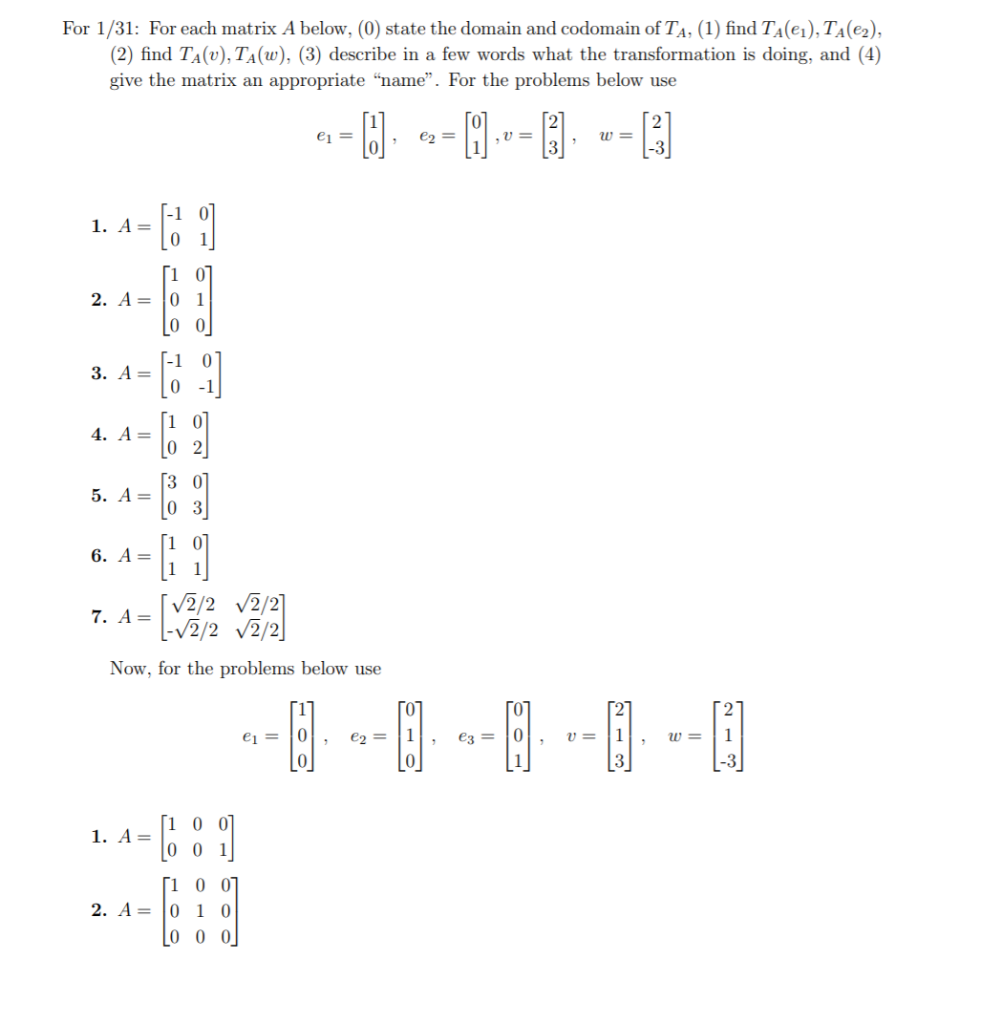Domain And Codomain Of A Matrix Transformation

The previous three examples can be summarized as follows. If you compute a nonzero vector v in the null space by row reducing and finding. This means that the null space of a is not the zero space.

domain and hosting tutorial domain and hosting in kenya domain and hosting business domain and hosting renewal domain and hosting emails domain and range guided notes algebra 1 domain and hosting bundle domain and hosting in nigeria

Matrix Transformations 1 4 Domain Codomain Range Standard Matrix Passing Linear Algebra Youtube

Linear Algebra Domain And Codomain Of A Matrix Transformation Homeworkhelp

Pin On Math Notes Jee Neet

Solved 7 Find The Standard Matrix Domain And Codomain Chegg Com

Solved For Each Matrix A Define The Linear Transformatio Chegg Com

Solved The Matrix A Is Row Equivalent To K Define A Li Chegg Com

The codomain is the set of values that could possibly come out.

Domain and codomain of a matrix transformation. Reflection dilation rotation shear projection. Where a is the transformation matrix x is a vector in the domain and y is the resulting vector in the codomain. Linear trans formations math 240. All of the vectors in the null space are solutions to t x 0.

If matrix a has 5 columns and 4 rows then by recalling the rules of matrix multiplication we know that x must have 5 rows and 1 column and that the result of the multiplication must have 4 rows and 1 column. Examples i t. And the range is the set of values that actually do come out. This means that applying the transformation t to a vector is the same as multiplying by this matrix.

Instead we simply plug the basis vectors into the transformation and then determine how to write the output in terms of the basis of the codomain. Domain and w the codomain of t. The codomain is actually part of the definition of the function. By the theorem there is a nontrivial solution of ax 0.

The codomain and range are both on the output side but are subtly different. The transformation uses matrix multiplication to turn a vector x with 4 elements into a vector with 6. I the range of a linear transformation is a subspace of its codomain. I the range of a matrix transformation is the column space of the matrix.

The matrix of a linear transformation is a matrix for which t vec x a vec x for a vector vec x in the domain of t. The domain is r 4 and the codomain r 6. Understand the domain codomain and range of a matrix transformation. Rn rm de ned by t x ax where a is an.

The matrix a is 4x6. Learn examples of matrix transformations. Learn to view a matrix geometrically as a function. When we compute the matrix of a transformation with respect to a non standard basis we don t have to worry about how to write vectors in the domain in terms of that basis.

Understand the vocabulary surrounding transformations. I don t know what it means by an image so i can t help out. Suppose that t x ax is a matrix transformation that is not one to one.