Domain And Range Of Y Radical X

This video provided examples of how to find the domain and range of radical functions with index 2 3 and 4.

Domain and range of y radical x. To find the range is a bit tricky. The range of a function f x is the set of all values of f x where x is in the domain of f. The example below shows two different ways that a function can be represented. Arithmetic mean geometric mean quadratic mean median mode order minimum maximum probability mid range range standard deviation variance lower quartile upper quartile interquartile range midhinge.

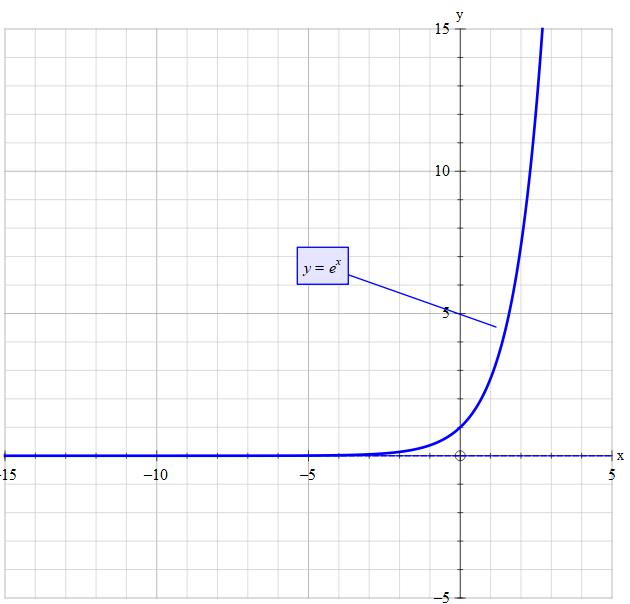
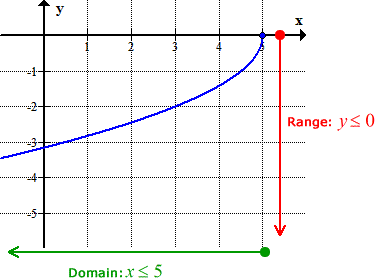
So far we have been able to find the inverse functions of cubic functions without having to restrict their domains. The domain and range are easy to find for this function. The domain and range of a function is all the possible values of the independent variable x for which y is defined. Find the domain and range y square root of x y x y x set the radicand in x x greater than or equal to 0 0 to find where the expression is defined.

Here our domain is all x values but does not include x 2. Find the domain and range y square root of x 7 y x 7 y x 7 set the radicand in x 7 x 7 greater than or equal to 0 0 to find where the expression is defined. Plugging in this domain into the function f x will also have all the values greater. The graph below shows that x 2 is actually a vertical asymptote see the dashed orange line.

As a function table and as a set of coordinates. For even numbered radical functions the term inside the radical must be at or above zero otherwise it is undefined. However as we know not all cubic polynomials are one to one. The results are checked graphically site.

X 7 0 x 7 0. Since x is the only thing inside the radical x itself must be greater than or equal to zero. This includes square roots. A linear function or b quadratic function domain and range of a radical function radical function is any function that contains a variable inside a root.

Domain and range of a radical function range of a function f x the radicand can either be. It makes a lot of sense because i can plug any values of x into the function with the exception of x 2 and the function will have valid outputs. The domain of a function f x is the set of all values of x for which f x is defined. For odd numbered radicals both the domain and range span all real number.

Some functions that are not one to one may have their domain restricted so that they are one to one but only over that domain. Chemical reactions chemical properties.