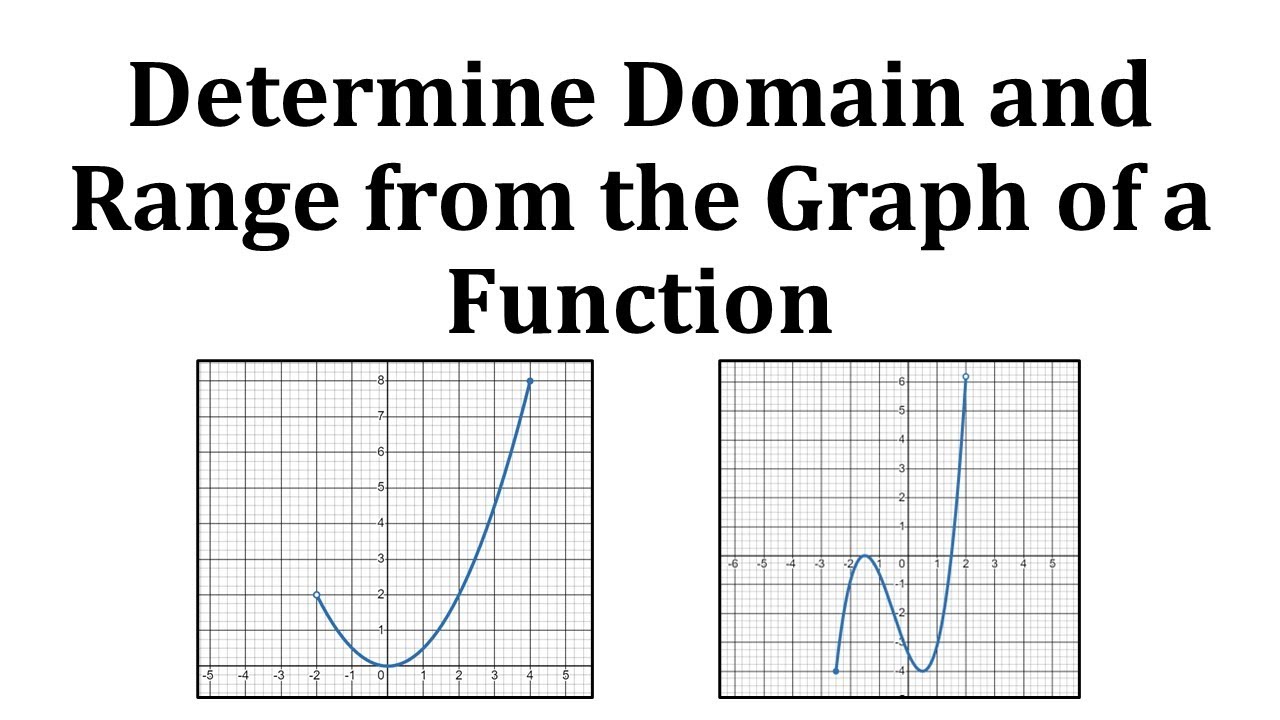
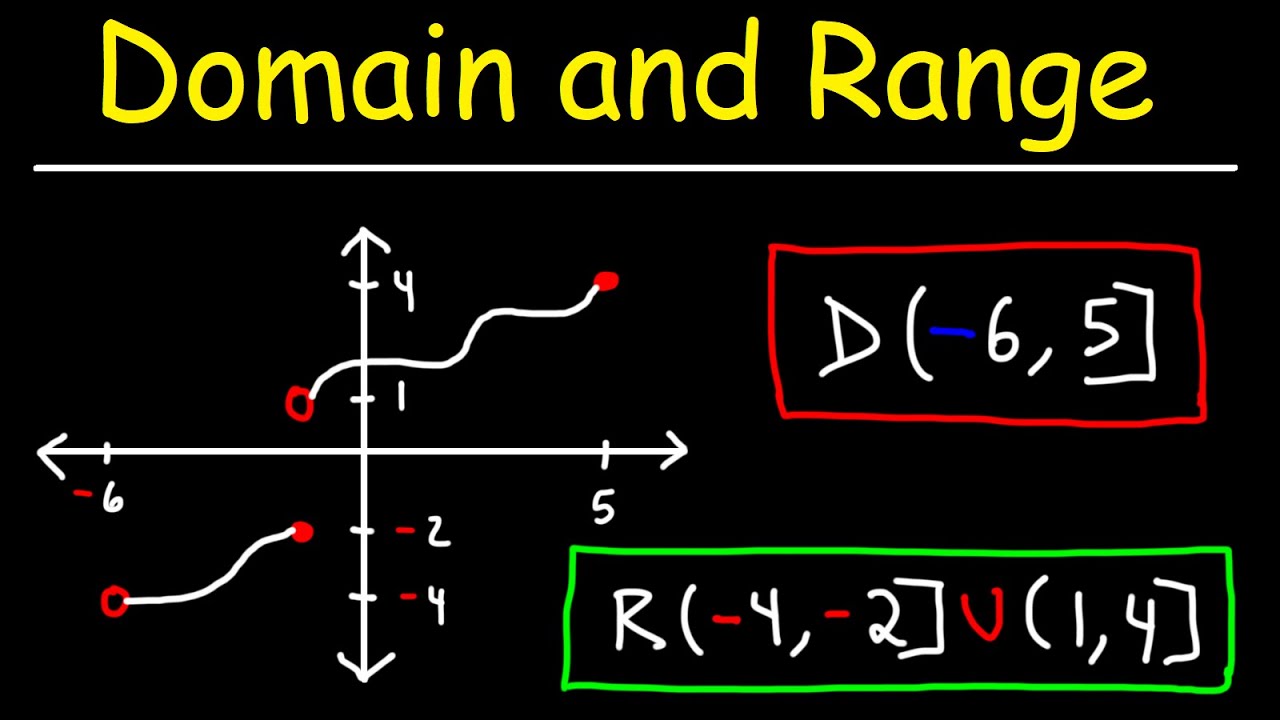
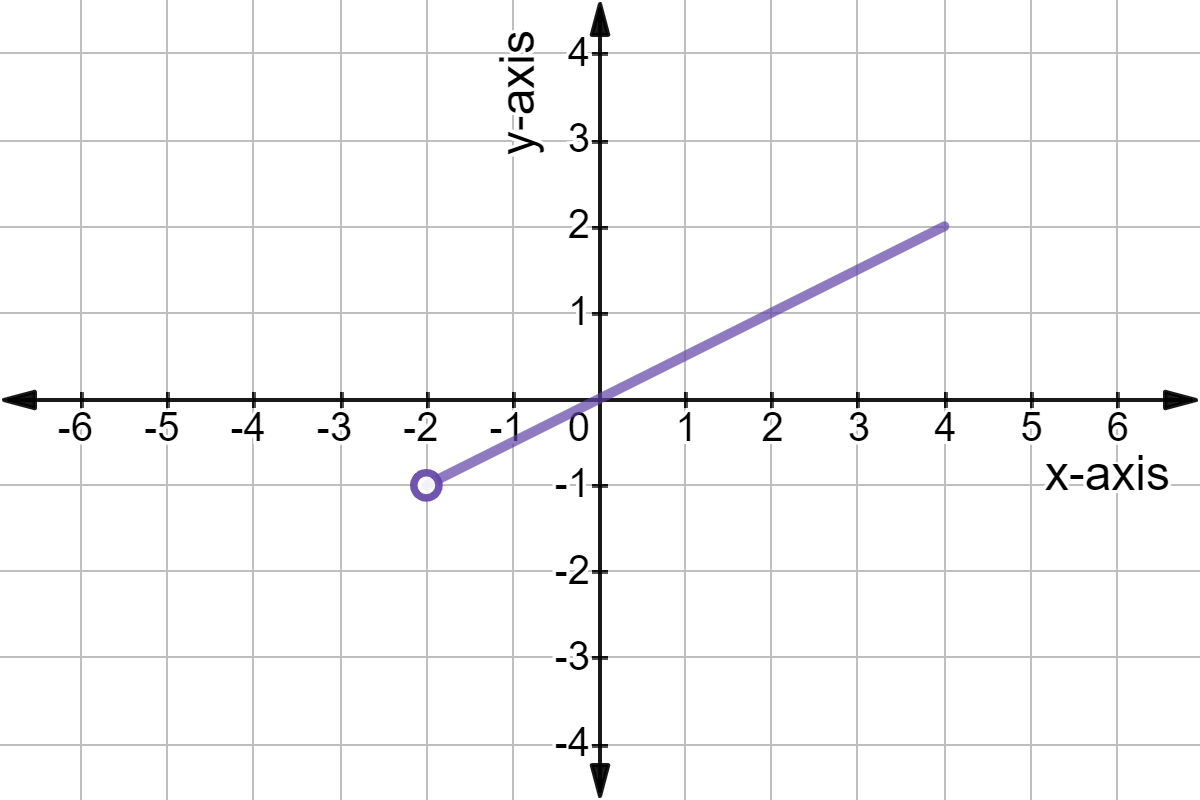
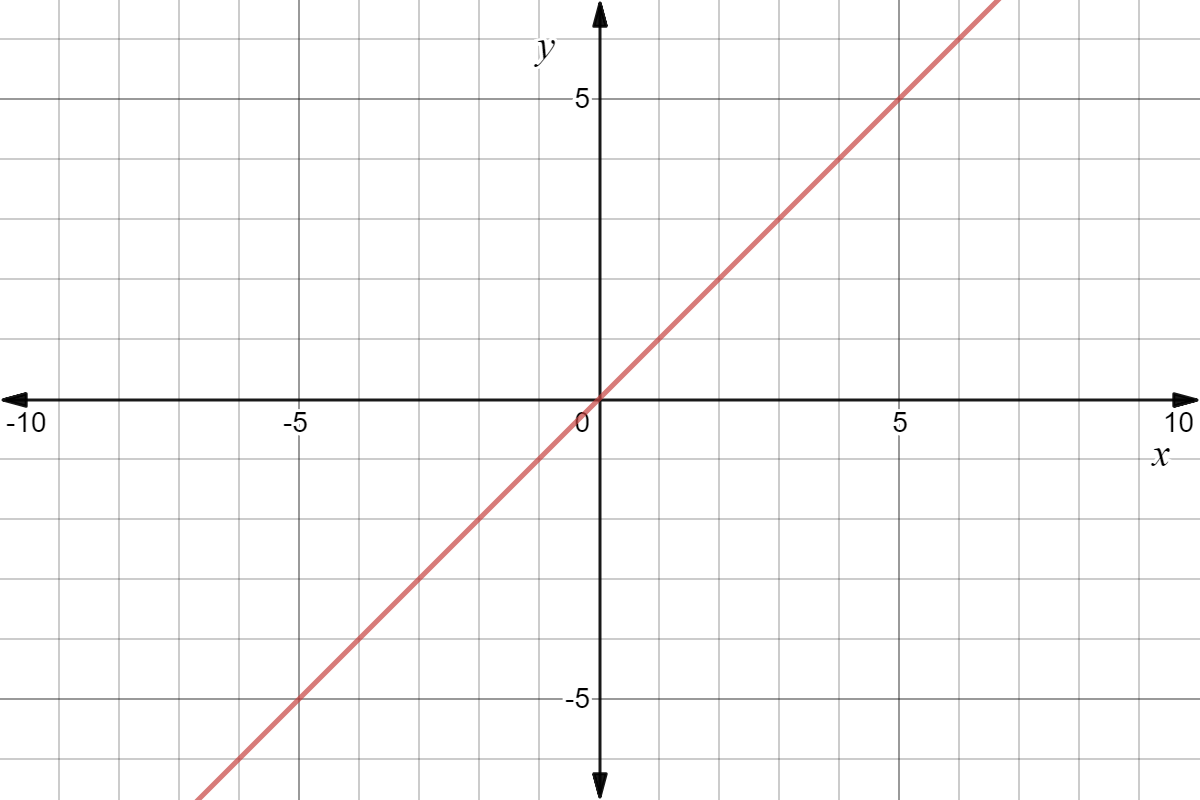
Using Interval Notation Identify The Domain And Range Of Each Graphed Function Below

10 2 s 8 10 123 5.

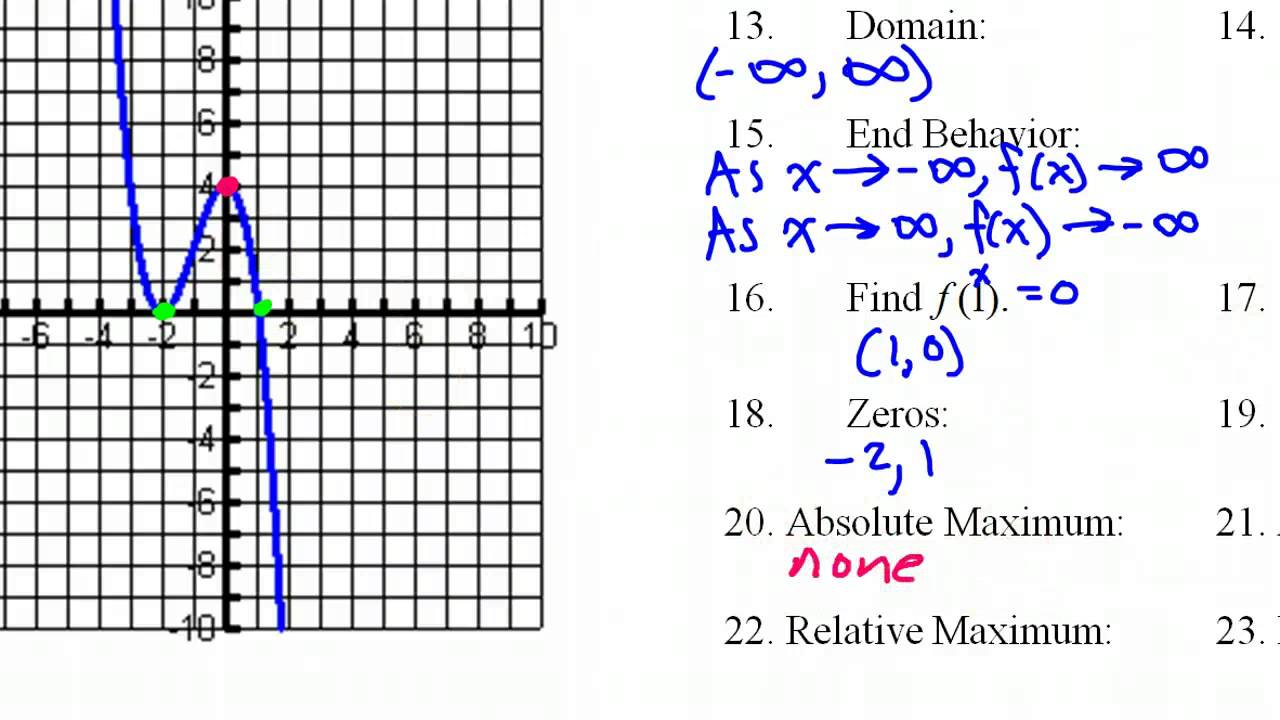
Using interval notation identify the domain and range of each graphed function below. Because the domain refers to the set of possible input values the domain of a graph consists of all the input values shown on the x axis. 8 2 1 2 2 6 3 8 4 4 3 2 1 0 1 4 3 4 is 4 10 2 o o 3 2 o 2 name the domain and range of each relation using interval notation. The domain and range of a function is all the possible values of the independent variable x for which y is defined. The range is the set of possible output values which are shown on the y axis.

Thus the domain of the function is the interval that means all the real number less than or equal to 4. Therefore the domain for the function is. For each number line write the given set of numbers in interval notation. When looking at a graph the domain is all the values of the graph from left to right.

The example below shows two different ways that a function can be represented. The range of the function is the subset of the co domain. Keep in mind that if the graph continues beyond the portion of the graph we can see the domain and range may be greater than the visible values. The range is the interval 2 the range is all real numbers greater than or equal to.

This makes the range y 0. The range is all the values of the graph from down to up. The domain calculator allows you to take a simple or complex function and find the domain in both interval and set notation instantly. Another way to identify the domain and range of functions is by using graphs.

Generally for continuous linear function equations the domain and range will be all real numbers except when the denominator becomes zero 0. The elements of the range represents on the axis and these. Below is the summary of both domain and range. The range of the function is the set of all output value of the function.

Click the blue arrow to submit and see the. Domain of a function calculator. The range of a function is all the possible values of the dependent variable y. Domain of a function calculator.

Identify which of the toolkit functions besides the quadratic function are not one to one and find a restricted domain on which each function is one to one if any. The domain is all x values or inputs of a function and the range is all y values or outputs of a function. This video will help you to understand the basic concepts and methods for deriving the domain range and some of the interval notations used for non continuous functions. Enter the function you want to domain into the editor.

We restrict the domain in such a fashion that the function assumes all y values exactly once. The vertex of the function is the point. As a function table and as a set of coordinates. The parabola open upward the vertex is a minimum.